Rigid body; Degrees of freedom, Euler’s theorem, angular velocity, angular momentum, moments of inertia, theorems of parallel and perpendicular axes,
Questions:
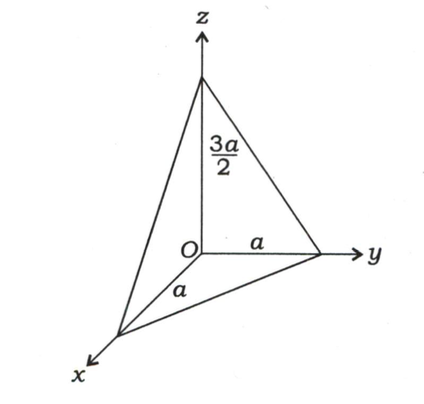
Q 1. A homogeneous right triangular pyramid wih the base side and height is shown below. Obtain the moment of inertia tensor of the pyramid. [15 marks]
Q 2. The angular momentum of a rigid body comprising of N particles and rotating with angular velocity is given by , where the origin coincides with the centre of mass. Express the components of in terms of components of the inertia tensor. Hence, show that the most general free rotation of a spherical top is a uniform rotation about an axis fixed in space. [15 marks]
Video Solution: